Bài 4.14 trang 58

Đề bài

Cho tam giác ABC

a) Hãy xác định điểm M để \(\overrightarrow {MA}  + \overrightarrow {MB}  + 2\overrightarrow {MC}  = \overrightarrow 0 \)

b) Chứng minh rằng với mọi điểm O, ta có \(\overrightarrow {OA}  + \overrightarrow {OB}  + 2\overrightarrow {OC}  = 4\overrightarrow {OM} \)

Lời giải chi tiết

a) Ta có: \(\overrightarrow {MA}  + \overrightarrow {MB}  + 2\overrightarrow {MC}  = \overrightarrow 0  \Leftrightarrow \overrightarrow {MA}  + \left( {\overrightarrow {MA}  + \overrightarrow {AB} } \right) + 2\left( {\overrightarrow {MA}  + \overrightarrow {AC} } \right) = \overrightarrow 0 \)

\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MA}  + \overrightarrow {AB}  + 2\overrightarrow {AC}  = \overrightarrow 0 \\ \Leftrightarrow 4\overrightarrow {AM}  = \overrightarrow {AB}  + 2\overrightarrow {AC} \\ \Leftrightarrow \overrightarrow {AM}  = \frac{1}{4}\overrightarrow {AB}  + \frac{1}{2}\overrightarrow {AC} \end{array}\)

Trên cạnh AB, AC lấy điểm D, E sao cho \(AD = \frac{1}{4}AB;\;\,AE = \frac{1}{2}AC\)

 

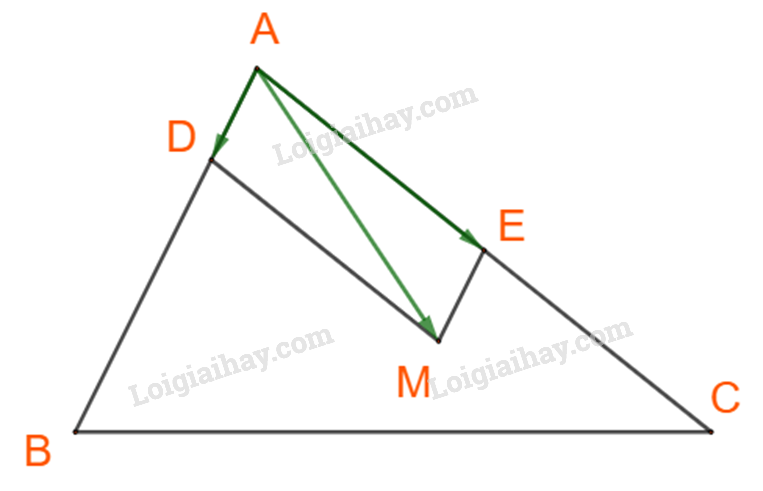
Khi đó \(\overrightarrow {AM}  = \overrightarrow {AD}  + \overrightarrow {AE} \) hay M là đỉnh thứ tư của hình bình hành AEMD.

 

Cách 2:

Ta có: \(\overrightarrow {MA}  + \overrightarrow {MB}  + 2\overrightarrow {MC}  = \overrightarrow 0  \Leftrightarrow \left( {\overrightarrow {MC}  + \overrightarrow {CA} } \right) + \left( {\overrightarrow {MC}  + \overrightarrow {CB} } \right) + 2\overrightarrow {MC}  = \overrightarrow 0 \)

\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MC}  + \overrightarrow {CA}  + \overrightarrow {CB}  = \overrightarrow 0 \\ \Leftrightarrow 4.\overrightarrow {CM}  = \overrightarrow {CA}  + \overrightarrow {CB} \end{array}\)

Gọi D là đỉnh thứ tư của hình bình hành ACBD.

Khi đó: \(\overrightarrow {CD}  = \overrightarrow {CA}  + \overrightarrow {CB} \)\( \Rightarrow 4.\overrightarrow {CM}  = \overrightarrow {CD} \)

\( \Leftrightarrow \overrightarrow {CM}  = \frac{1}{4}\overrightarrow {CD}  \Leftrightarrow \overrightarrow {CM}  = \frac{1}{2}\overrightarrow {CO} \)

Với O là tâm hình bình hành ACBD, cũng là trung điểm đoạn AB.

 

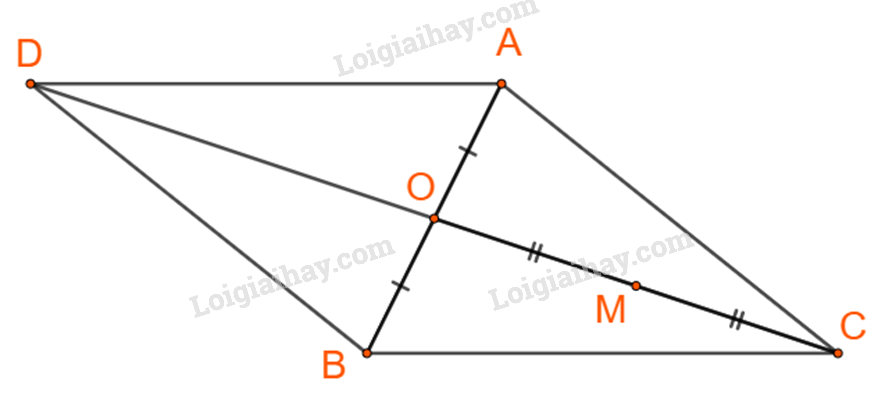
Vậy M là trung điểm của trung tuyến kẻ từ C của tam giác ABC.

b) Chứng minh rằng với mọi điểm O, ta có \(\overrightarrow {OA}  + \overrightarrow {OB}  + 2\overrightarrow {OC}  = 4\overrightarrow {OM} \)

Với mọi điểm O, ta có: \(\left\{ \begin{array}{l}\overrightarrow {OA}  = \overrightarrow {OM}  + \overrightarrow {MA} ;\;\\\overrightarrow {OB}  = \overrightarrow {OM}  + \overrightarrow {MB} ;\;\,\\\overrightarrow {OC}  = \overrightarrow {OM}  + \overrightarrow {MC} \end{array} \right.\)

\(\begin{array}{l} \Rightarrow \overrightarrow {OA}  + \overrightarrow {OB}  + 2\overrightarrow {OC}  = \left( {\overrightarrow {OM}  + \overrightarrow {MA} } \right) + \left( {\overrightarrow {OM}  + \overrightarrow {MB} } \right) + 2\left( {\overrightarrow {OM}  + \overrightarrow {MC} } \right)\\ = 4\overrightarrow {OM}  + \left( {\overrightarrow {MA}  + \overrightarrow {MB}  + 2\overrightarrow {MC} } \right) = 4\overrightarrow {OM}  + \overrightarrow 0  = 4\overrightarrow {OM} .\end{array}\)

Vậy với mọi điểm O, ta có \(\overrightarrow {OA}  + \overrightarrow {OB}  + 2\overrightarrow {OC}  = 4\overrightarrow {OM} \).