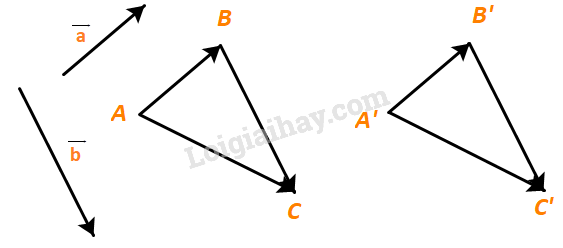
HĐ1
Với hai vectơ \(\overrightarrow a ,\overrightarrow b \) cho trước, lấy một điểm A vẽ các vectơ \(\overrightarrow {AB} = \overrightarrow a ,\;\overrightarrow {BC} = \overrightarrow b \). Lấy điểm A’ khác A và cũng vẽ các vectơ \(\overrightarrow {A'B'} = \overrightarrow a ,\;\overrightarrow {B'C'} = \overrightarrow b \). Hỏi hai vectơ \(\overrightarrow {AC} \) và \(\overrightarrow {A'C'} \) có mối quan hệ gì?
Phương pháp giải:
Hai vectơ bằng nhau nếu chúng có cùng độ dài và cùng hướng.
Xét độ dài và hướng của hai vectơ \(\overrightarrow {AC} \) và \(\overrightarrow {A'C'} \) để suy ra mối quan hệ của chúng.
Lời giải chi tiết:
\(\overrightarrow {AB} = \overrightarrow a \;\;\, \Rightarrow \left\{ \begin{array}{l}AB//\;a\\AB = a\end{array} \right.\) và \(\overrightarrow {A'B'} = \overrightarrow a \;\;\, \Rightarrow \left\{ \begin{array}{l}A'B'\;//\;a\\A'B' = a\end{array} \right.\)
\( \Rightarrow \left\{ \begin{array}{l}AB//\;A'B'\\AB = A'B'\end{array} \right.\)
Tương tự, ta cũng suy ra \(\left\{ \begin{array}{l}BC//\;B'C'\\BC = B'C'\end{array} \right.\)
\( \Rightarrow \Delta ABC = \Delta A'B'C'\)(c-g-c)
\(\left\{ \begin{array}{l}AC//\;A'C'\\AC = A'C'\end{array} \right.\)
Dễ dàng suy ra \(\overrightarrow {AC} = \overrightarrow {A'C'} \).
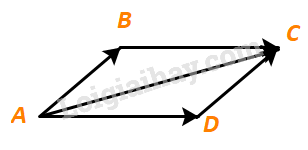
HĐ2
Cho hình bình hành ABCD. Tìm mối quan hệ giữa hai vectơ \(\overrightarrow {AB} + \overrightarrow {AD} \) và \(\overrightarrow {AC} \)
Phương pháp giải:
Bước 1: Xác định vectơ \(\overrightarrow {AB} + \overrightarrow {AD} \) bằng cách thay vectơ \(\overrightarrow {AD} \) bởi vectơ bằng nó mà có điểm đầu là B.
Bước 2: So sánh với vectơ \(\overrightarrow {AC} \)
Lời giải chi tiết:
Vì ABCD là hình bình hành nên \(\left\{ \begin{array}{l}AD//\;BC\\AD = BC\end{array} \right.\), hay \(\overrightarrow {AD} = \overrightarrow {BC} \).
Do đó \(\overrightarrow {AB} + \overrightarrow {AD} = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \).
HĐ3
a) Trong hình 4.14a, hãy chỉ ra vectơ \(\overrightarrow a + \overrightarrow b \)và vectơ \(\overrightarrow b + \overrightarrow a \).
b) Trong hình 4.14b, hãy chỉ ra vectơ \(\left( {\overrightarrow a + \overrightarrow b } \right) + \overrightarrow c \)và vectơ \(\overrightarrow a + \left( {\overrightarrow b + \overrightarrow c } \right)\).

Phương pháp giải:
Nếu \(\overrightarrow {AB} = \overrightarrow a ,\;\overrightarrow {BC} = \overrightarrow b \) thì \(\overrightarrow a + \overrightarrow b = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)
Lời giải chi tiết:
a) Ta có: \(\overrightarrow {AB} = \overrightarrow a ,\;\overrightarrow {BC} = \overrightarrow b \) nên \(\overrightarrow a + \overrightarrow b = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \)
Mặt khác: \(\overrightarrow {AD} = \overrightarrow b ,\;\overrightarrow {DC} = \overrightarrow a \) nên \(\overrightarrow b + \overrightarrow a = \overrightarrow {AD} + \overrightarrow {DC} = \overrightarrow {AC} \)
Do đó \(\overrightarrow a + \overrightarrow b = \overrightarrow b + \overrightarrow a \).
b) Theo câu a) ta có \(\overrightarrow a + \overrightarrow b = \overrightarrow {AC} \) và \(\overrightarrow {CD} = \overrightarrow c \) nên \(\left( {\overrightarrow a + \overrightarrow b } \right) + \overrightarrow c = \overrightarrow {AC} + \overrightarrow {CD} = \overrightarrow {AD} \).
Mặt khác: \(\overrightarrow {BC} = \overrightarrow b ,\;\overrightarrow {CD} = \overrightarrow c \) nên \(\overrightarrow b + \overrightarrow c = \overrightarrow {BC} + \overrightarrow {CD} = \overrightarrow {BD} \)
Và \(\overrightarrow a = \overrightarrow {AB} \) nên \(\overrightarrow a + \left( {\overrightarrow b + \overrightarrow c } \right) = \overrightarrow {AB} + \overrightarrow {BD} = \overrightarrow {AD} \)
Vậy \(\left( {\overrightarrow a + \overrightarrow b } \right) + \overrightarrow c = \overrightarrow a + \left( {\overrightarrow b + \overrightarrow c } \right)\)
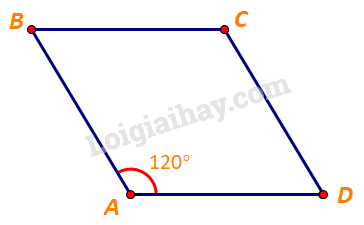
Luyện tập 1
Cho hình thoi ABCD cới cạnh có độ dài bằng 1 và \(\widehat {BAD} = {120^o}\). Tính độ dài của các vectơ \(\overrightarrow {CB} + \overrightarrow {CD} ,\;\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} .\)
Lời giải chi tiết:
\(\overrightarrow {CD} = \overrightarrow {BA} \) do hai vectơ \(\overrightarrow {CD} ,\;\overrightarrow {BA} \) cùng hướng và \(CD = BA\).
\(\begin{array}{l} \Rightarrow \overrightarrow {CB} + \overrightarrow {CD} = \overrightarrow {CB} + \overrightarrow {BA} = \overrightarrow {CA} \\ \Leftrightarrow \left| {\overrightarrow {CB} + \overrightarrow {CD} } \right| = \left| {\overrightarrow {CA} } \right| = CA\end{array}\)
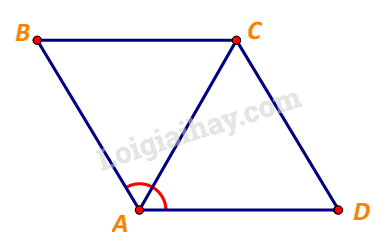
Xét tam giác ABC, ta có:
\(BA = BC\) và \(\widehat {BAC} = \frac{1}{2}.\widehat {BAD} = {60^o}\)
\( \Rightarrow \Delta ABC\) đều, hay \(CA = BC = 1\)
Vậy \(\left| {\overrightarrow {CB} + \overrightarrow {CD} } \right| = 1.\)
Dựa vào tính chất kết hợp, ta có:
\(\begin{array}{l}\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} = \left( {\overrightarrow {DB} + \overrightarrow {CD} } \right) + \overrightarrow {BA} \\ = \left( {\overrightarrow {CD} + \overrightarrow {DB} } \right) + \overrightarrow {BA} = \overrightarrow {CB} + \overrightarrow {BA} = \overrightarrow {CA} .\\ \Rightarrow \left| {\overrightarrow {DB} + \overrightarrow {CD} + \overrightarrow {BA} } \right| = \left| {\overrightarrow {CA} } \right| = CA = 1.\end{array}\)
